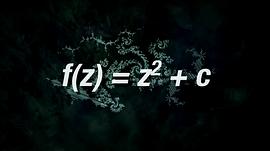以分形理论来说,fractal could be found in everywhere
那么时候地球所在的太阳系甚至银河系也是茫茫宇宙的某个分形中的一只branch?
神奇

Go with basic and simple purpose, conducting similarity and virable options which is callled EVOLUTION.
I do like it, from the smoothly math to the real change of the world around us. Similarity is not only a Math but sharing the same vision on physics, from Newton, to Einstein, to the parallel universe.

Loren Carpenter (visualize)-> what the planes might look like in flight.
Fractals - Form, Chance, and Dimension by Benoit Mandelbrot
It's one of the keys to fractal geometry call iteration in mathematicians.
First Mountain and then "Star Trek II" the Wrath of khan.
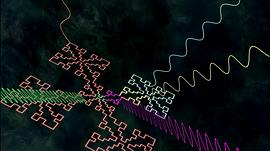
Self-similarity always zoom in and out the object look the same.
People like the great 19th century Japanese artist Katsushika Hokusai
the mystery of the monsters, a story really begins in later 19 century, Georg Cantor (German)
Created first monsters in 1883, call " Cantor Set."
Another by the Swedish Helge Von Koch, one of the classical Euclidean geometric figures.
in the 1940s, British Scientist Lewis Richardson,
Koch Curve he wrote a very famous article i Science Magazine called " How Long is the Coastline of British."
Dimension
French Gaston Julia
Mandelbrot in IBM

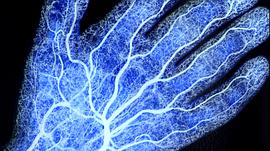
The film is about fractal geometry. Someone calls fractal geometry 'the natural dynamics of everything' (a video title, 2011, available at https://www.youtube.com/watch?v=yUM7e0tIFi0). Why? Because it explains the shapes of everything in the nature: why the British coastline looks like that, why mountains looks like that, why the trees look like that, why the vessels in the body look like that, ect., etc..
Fractal geometry was invented by Benoit Mandelbrot from 1950. In general, it is a combination of classical geometry (coined by Euclid) and algorithm. The most famous fractal - The Mandelbrot Set - derives from a circle and a generating function 'f(z) = z^2 + c'. (For more knowledge, visit http://mathworld.wolfram.com/MandelbrotSet.html)

曼德尔布洛特集
科赫雪花
分形应用
用于探知为何体积越大单位所需能量越少,即E=M的3/4方。
用于解决无线通讯中如蓝牙、无线通讯、wifi等需要单独频率但避免多个天线的应用
用于检测心脏健康。
三年前看过的纪录片,这是我——一个理科不好的同学对于自然科学最后的反扑,因为硬想要深刻所以觉得这部显得平淡,其实放开了心态看的话,就只要坐着感叹好美啊好美啊好奇妙啊就可以了,科学原理乃至现实应用不妨交给科学家们来做。

寻找隐秘的维度:Hunting the hidden dimension PBS
转载请注明网址: //m.keyitservice.com/dy/id-59835.html